
Nonlinear Oscillations in Mechanical Systems
(A Virtual Lab for Undergraduate and Graduate Students)
Various linear systems constitute the backbone of introductory physics because they are described by mathematically simple linear differential equations. In particular, the treatment of oscillations in traditional physics courses is restricted, as a rule, to various harmonic oscillatory systems, whose behavior can be almost completely investigated analytically by means of rather simple mathematics accessible for college and undergraduate university students. However, we should realize that such restrictions might lead students to a distorted conception of oscillatory physics as a whole. Detailed investigation of linear systems is certainly very important, but insufficient.
The periodically forced linear oscillator (with harmonic potential) gives only periodic motion of the same period in the steady-state response. Specifically, under sinusoidal driving force this steady-state motion is also purely sinusoidal its spectrum consists of the single (principal) harmonic whose frequency equals the driving frequency. Various anharmonic potentials that correspond to nonlinear restoring forces can lead to a great variety of different modes of transient and steady-state responses, including subharmonic and superharmonic resonances, hysteretic transient and chaotic steady-state behavior. Chaos, which is a type of essentially unpredictable behavior exhibited by a variety nonlinear deterministic systems, has been a subject of intense interest during recent years. In order to observe chaotic behavior, however, the equation of motion must be nonlinear. Numerical simulation becomes then an essential tool to aid understanding of the phenomenon.
Simulations provide of course just one way of allowing students to better understand science. However, simulations have special advantages. Simulations can produce images of considerable power that help students remember details in a way which equations cannot. Interactivity is also a powerful argument in favour of simulations. Students quickly become bored if they are just passive consumers of information. They learn better when they need to respond frequently to computer software. At the same time interactive software must be sufficiently structured that users do not lose sight of the educational target.
The package NONLINEAR OSCILLATIONS includes a set of highly interactive programs that allow you to observe the simulations of simple nonlinear mechanical oscillatory systems. You can obtain time-dependent graphs of the variables that describe the simulated system, phase diagrams and graphs of energy transformations. Graphs and diagrams appear on the screen simultaneously with the motion display. The suggested experiments have been designed to be plain and obvious. They provide the capability of observing repeatedly and thoroughly the fine details of phenomena that usually escape notice during direct observation. You can widely modify parameters of the investigated physical systems and conditions of the experiments. The graphic presentation of the results allows you to see and easily understand large amounts of information. The simulations bring to life many abstract concepts related to the physics of oscillations and can lead to considerable insight into the complex behavior exhibited by nonlinear systems.
The package NONLINEAR OSCILLATIONS can be helpful to a wide range of university students. The programs provide the instructor with powerful demonstration tools to accompany lectures in general physics and the theory of oscillations. With these programs, the students have an opportunity to perform interesting mini-research physics projects on their own.
In the package NONLINEAR OSCILLATIONS the following mechanical systems are simulated:
COMPUTER SYSTEM REQUIREMENTS:
Your hardware configuration should be any PC running under operating system Windows 98 or higher (Windows ME, Windows 2000, Windows XP). A high resolution screen mode (1024 x 768 or higher) is recommended. Printer is optional.
Click here (or on the button above) to download the archive file Nonlinear.zip (9.2 Mb) with the package NONLINEAR OSCILLATIONS. Then unzip it to a folder on your local machine and run a standard setup procedure (click on the file setup.exe). The setup program will copy the NONLINEAR OSCILLATIONS files to a folder on your hard disk and create an entry in the Start menu. To launch the simulation programs, click on the icon "Nonlinear Oscillations" created on your desktop by the setup procedure.
We note that all programs of the package are supplied with several sets of predefined examples illustrating many interesting modes of the simulated system behavior. To open the list of examples, click on the button "Demo Examples" on the title screen, or on the menu item "Examples" which can be found in the upper line of any screen, or in the pop-up menu called by clicking the right mouse button. We recommend to use these examples at first acquaintance with the programs. Additional background material explaining the simulated physical systems and different modes of their behavior can be found in text documents and articles (pdf files) that supplement almost all programs of the package. You will need Adobe Acrobat Reader program to read papers and other text documents of the package. These materials are available directly from the programs by clicking "Help on physics" in the menu. Some of the papers have been published in the literature:
As a suitable prerequisite for successful work with the package NONLINEAR OSCILLATIONS, we recommend the new edition of the award-winning educational software PHYSICS OF OSCILLATIONS. The package includes a set of highly interactive programs that allow the user to observe the simulations of simple mechanical oscillatory systems, and obtain time-dependent graphs of the variables that describe the simulated system, phase diagrams and graphs of energy transformations. Graphs and diagrams appear on the screen simultaneously with the display of motion. The suggested experiments have been designed to be plain and obvious. The user can widely modify parameters of the investigated physical systems and conditions of the experiments. The simulations bring to life many abstract concepts related to the physics of oscillations (see annotation). The package PHYSICS OF OSCILLATIONS is supplied with a 160-page Users Manual that gives a theoretical background for the simulated mechanical systems and suggests students' activities. The manual contains hundreds of problems and exercises. A 95-page Instructors Guide gives details of their solutions.
Last revised: 12. 04. 2006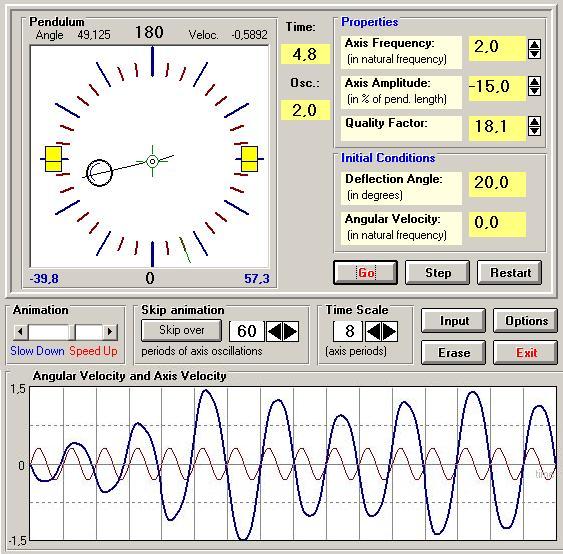
The project NONLINEAR OSCILLATIONS is not yet finalized: simulations of some other nonlinear systems will be added in the future versions, as well as some new or improved papers with theoretical investigations of the simulated systems.

Nonlinear Oscillations a Virtual Lab